Closed-Loop Design

From Basic Layer Information to Input-Output Characteristics

The following example demonstrates the predictive capabilities and accuracy of the microscopic models used to set up the gain databases. It shows how the databases can be used to predict fundamental operating characteristics of operating devices using only basic information that is usually available in an on-wafer stage of the development.

This example is outlined in more detail in Ref.[30]. Some additional information about this example and general capabilities of the microscopic models can be found in Ref.[35].

The structure is a ridge Fabry-Perot laser containing of four 6nm wide In_0.9Ga_0.1As_0.26P_0.74/InP quantum wells. The doping profile has been calculated to result in an internal electric field across the active region of 80 kV/cm. The ridge is 3.8µm wide and 750µm long. From the reflectivities of the cleaved facets the outcoupling loss is calculated to be 17.2/cm. Measurements of the threshold of devices of varying lengths yielded an internal loss of 10.6/cm. The confinement factor is 0.0263.



Spontaneous Emission Analysis


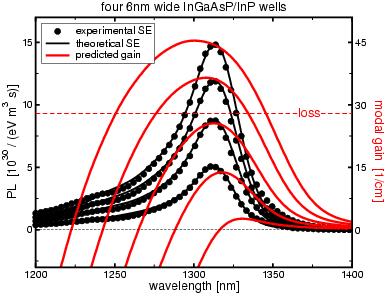
In the first step the spontaneous emission (PL) of the un-processed sample is measured for several excitation densities in the low density regime. The results are shown as black dots in the picture above. As described in the PL Analysis example and Ref.[15], these spectra are compared to theoretical ones in order to determine the inhomogeneous broadening (here: 14meV FWHM) and possible deviations from the nominal structural design. The theoretical spectra have to be shifted by -23nm corresponding to a slightly smaller Indium- or Arsenic-concentration in the well than nominal.
The corresponding spontaneous emission spectra are shown as black lines in the picture above. Also shown in that picture as red lines are theoretical gain spectra for several carrier densities after applying the determined inhomogeneous broadening and spectral shift.



Calculating Loss Currents



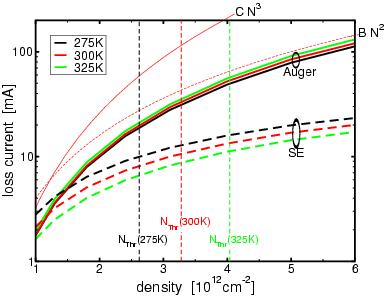
Using the fit-parameter free fully microscopic models described in detail in Ref.[28] the loss currents due to radiative recombination and Auger processes are calculated for various carrier densities. the results are shown in the picture above for three temperatures. Also shown in that picture as thin red lines are loss currents for 300K when using the power laws BN² and CN³ for the radiative and Auger losses, respectively. Here, the coefficients B and C have been determined from a fit to the microscopic results at very low densities where the power laws are known to be fairly correct. Despite knowing the correct low-density coefficients, these laws obviously overestimate dramatically the loss currents in the density regime relevant for laser operation.
The threshold carrier density, N_thr, is determined by looking up from the gain table the density that yields enough gain after inhomogeneous broadening to overcome the outcoupling and internal losses. The corresponding densities are marked by dashed vertical lines in the above picture.



Comparison to the Experiment



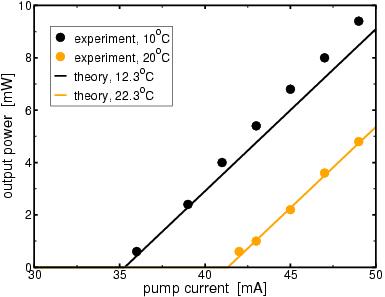
The picture above shows the experimental input-output characteristic for this device at two temperatures (dots) together with what the theory would predict (lines). The theoretical threshold is the sum of the Auger and radiative loss currents. Defect recombination is assumed to be negligible in this high quality MBE-grown structure. The slope of the characteristics is given by the ratio between outcoupling loss and total loss.
Assuming the nominal experimental tmperature the theoretical thresholds are about 1.4mA too small. This slight mismatch is probably due to some internal heating during operation. Assuming a 2.3K higher temperature than nominal excellent agreement between theory is obtained.

It is worth stressing that these theoretical results do not include any adjustable parameters. Of course, when using e.g. an Auger parameter, C, and its temperature dependence as adjustable fit parameters, a good fit to experimental data as shown here can easily achieved. However, such a fit requires previous knowledge of the experimental result and has no predictive capabilities. Even trying to extend the analysis by using the fitted Auger coefficients will generally fail due to the sensitivity of the underlying processes to any variables like temperature, density or structural variations.

In contrast, the only information used here is the nominal structural layout, the information about the internal losses, the low excitation spontaneous emission spectra and general material parameters from the standard literature.